Quantum Physics

A photon is a discrete packet {or quantum} of energy of an electromagnetic radiation/wave.

Energy of a photon, E = h f = hc / λ where h: Planck's constant

λ_violet ≈ 4 x 10^-7 m, λ_red ≈ 7 x 10^-7 m

Power of electromagnetic radiation, P = Rate of incidence of photon x Energy of a photon = (N/t)(hc/λ)

Photoelectric effect refers to the emission of electrons from a cold metal surface when electromagnetic radiation of sufficiently high frequency falls on it.

4 Major Observations:

1. No electrons are emitted if the frequency of the light is below a minimum frequency {called the threshold frequency}, regardless of the intensity of light
2. Rate of electron emission {ie photoelectric current} is proportional to the light intensity.
3. {Emitted electrons have a range of kinetic energy, ranging from zero to a certain maximum value. Increasing the freq increases the kinetic energies of the emitted electrons and in particular, increases the maximum kinetic energy.} This maximum kinetic energy depends only on the frequency and the metal used {ϕ}; the intensity has no effect on the kinetic energy of the electrons.
4. Emission of electrons begins instantaneously {i.e. no time lag between emission & illumination} even if the intensity is very low.
   NB: (1), (3) & (4) cannot be explained by Wave Theory of Light; instead they provide evidence for the particulate/particle nature of electromagnetic radiation.

Explanation for how photoelectric effect provides evidence for the particulate nature of em radiation:
{Consider the observations (1), (3) & (4). Use any 2 observations above to describe how they provide evidence that em radiation has a particle nature.}

• According to the “Particle Theory of Light”, em radiation consists of a stream of particles/photons/discrete energy packets, each of energy hf. Also, no more than one electron can absorb the energy of one photon {“All-or- Nothing Law”.}
• Thus if the energy of a photon hf < the minimum energy required for emission (ϕ), no emission can take place no matter how intense the light may be. {Explains observation (1)}
• This also explains why, {even at very low intensities}, as long as hf > ϕ, emission takes place without a time delay between illumination of the metal & ejection of electrons {Explains observation (4)}.

Threshold frequency is the minimum frequency of the em radiation required to eject an electron from a metal surface. {This is because the electrons are held back by the attractive forces of the positive nuclei in the metal.}

Work function of a metal is the minimum energy required to eject an electron from a metal surface

ϕ = h f0 = hcλ0	f0 = threshold frequency
	λ0 = threshold wavelength Maximum

Maximum KE of electrons, ½ m_ev²_max = eV_s {in magnitude} , Vs: stopping potential
hf = ϕ + eV_s

From eV_s = hf - ϕ

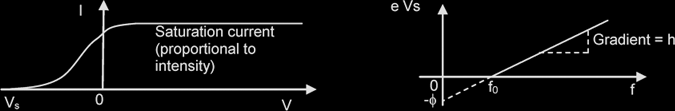
• If only intensity doubles, the saturation current doubles (V_s: no change)
• If only frequency increases, magnitude of V_s also increases, thus no change to saturation current.

Intensity = Incident Power / Illuminated Area = (N/t)(hc/λ)(1/Area)

Thereby Intensity ∝ Rate of incidence of photons, N/t {for a given λ}

Photocurrent I = (n/t)e, where (n/t) = rate of emission of electrons

Why rate of emission of electrons << rate of incidence of photons {for f>f₀}:

• Not every photon would collide with an electron; most are reflected by the metal or miss hitting any electron.
• On the way out to the metal surface, an electron may lose its kinetic energy to ions and other electrons it encounters along the way. This energy loss prevents it from overcoming the work function.

1 eV = (1.6 x 10^-19C)x (1V) = 1.6 x 10^-19J {Using W = QV}
1 nanometre (nm) = 1 x 10^-9m

Photoelectric equation: Energy of photon = Work function (energy) + Max. KE of electrons

hf = ϕ + ½ m_ev²_max

Wave-Particle Duality Concept

• Refers to the idea that light and matter {such as electrons} have both wave & particle properties.
• The wavelength of an object is given by λ = h/p {p: momentum of the particle.}
• Interference and diffraction provide evidence for the wave nature of E.M. radiation.
• Photoelectric effect provides evidence for the particulate nature of E.M. radiation.
• These evidences led to the concept of the wave-particle duality of light.

Electron diffraction provides evidence that matter / particles have also a wave nature & thus, have a dual nature.

de Broglie wavelength of a particle {“matter waves”}, λ = hp

Energy Levels of Isolated Atom:

• Are discrete {i.e. can only have certain energy values.}
• Difference between successive energy levels ΔE: decreases as we move from ground state upwards.

Explain how existence of electron energy levels in atoms gives rise to line spectra:

• Energy levels are discrete.
• During a downward transition, a photon is emitted.
• Freq of photon f = (E_i - E_f) / h
• Since E_i & E_f can only have discrete values, the freq are also discrete and so a line {rather than a continuous} spectrum is produced. {No need to mention role of spectrometer}

2 common ways to cause Excitation of an atom:

• When bombarded by an incident electron where KE of incident electron > ΔE
  i.e. (½ m_eu²)_{before collision} = Δ E + (½ m_ev²)_{after collision}
• Absorbing an incident photon of frequency f where hf must = ΔE exactly

The energy level of the ground state gives the ionization energy, i.e. the energy needed to completely remove an electron initially in the ground state from the atom {i.e. to the energy level n = ∞, where E_∞ =0}.

Emission line spectrum: A series of discrete/separate bright lines on a dark background, produced by electron transitions within an atom from higher to lower energy levels and emitting photons.

An excited atom during a downward transition emits a photon of frequency f, such that E_i - E_f = hf

Absorption line spectrum: A continuous bright spectrum crossed by “dark” lines. It is produced when “white light” passes through a cool gas. Atoms/electrons of the cool gas absorb photons of certain frequencies and get excited to higher energy levels which are then quickly re-emitted in all directions.

Characteristic X-rays: produced when an electron is knocked out of an inner shell of a target metal atom, allowing another electron from a higher energy level to drop down to fill the vacancy. The x-rays emitted have specific wavelengths, determined by the discrete energy levels which are characteristic of the target atom.

Continuous X-ray Spectrum {Braking Radiation (Bremsstrahlung)}: produced when electrons are suddenly decelerated upon collision with atoms of the metal target.

Minimum λ of cont. spectrum λ_min: given by hc / λ_min = eV_a , V_a: accelerating pd of x-ray tube

Heisenberg Uncertainty Principles: If a measurement of the position of a particle is made with uncertainty Δx and a simultaneous measurement of its momentum is made with uncertainty Δp, the product of these 2 uncertainties can never be smaller than h/4π

Δx Δp ≥ h / 4π

Similarly ΔE Δt ≥ h/4π where E is the energy of a particle at time t

A particle can be described by a wave function Ψ where the square of the amplitude of wave function, IΨ I², is proportional to the probability of finding the particle at a point.

Potential barrier: A region of electric field that prevents an atomic particle like an electron on one side of the barrier from passing through to the other side.

OR

• A region where the potential energy of a particle, if it is placed there, is greater than the total energy of the particle.
• Hence the particle would experience an opposing force if it tries to enter into the potential barrier

Quantum tunnelling: A quantum-mechanical process whereby a particle penetrates a classically forbidden region of space, i.e. the particle goes through a potential barrier even though it does not have enough energy to overcome it. Due to the wave nature of a particle, there is a non-zero probability that the particle is able to penetrate the potential barrier.

Scanning tunnelling microscope: Involves passing electrons from the tip of a probe through a potential barrier to a material that is to be scanned.

• Quantum tunnelling allows electrons to overcome the potential barrier between tip & material
• Magnitude of tunnelling current is dependent on the dist betw the tip and the surface.
• There are two methods to obtain images of the surface of the material:
  1. Maintain the tip at constant height and measure the tunnelling current
  2. Maintain a constant tunnelling current and measure the (vertical) position of the tip.

A feedback device adjusts the vertical height of the tip to keep the tunnelling current const as the tip is scanned over the surface {Method 2}). The output of the device provides an image of the surface contour of the material. )

Transmission coefficient (T): measures the probability of a particle tunnelling through a barrier.

T = e -2 k d	k = √[(8π2m(U - E))h2]
	d: the thickness of the barrier in metres
	m: mass of the tunnelling particle in kg
	U: the “height” of the potential barrier in J {NOT: eV}
	E: the energy of the electron in J
	h: The Planck's constant

Reflection coefficient (R): measures the probability that a particle gets reflected by a barrier.

T + R = 1

This temporary old revision section will automatically redirect to new version once ready.