Home Revision A-Level Physics D.C. Circuits

D.C. Circuits

Circuit Symbols:


Open Switch
Closed Switch
Lamp
Cell
Battery
Voltmeter

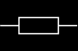Resistor
Fuse
Ammeter
Variable resistor
Thermistor
Light dependent resistor (LDR)

Resistors in Series: R = R1 + R2 + ...
Resistors in Parallel: 1/R = 1/R1 + 1/R2 + …

Example 1:
Three resistors of resistance 2 Ω, 3 Ω and 4 Ω respectively are used to make the combinations X, Y and Z shown in the diagrams. List the combinations in order of increasing resistance.

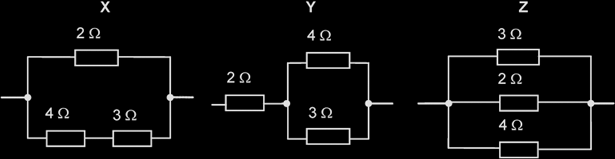
Resistance for X = [1/2 + 1/(4+3)]-1 = 1.56 Ω
Resistance for Y = 2 + (1/4 + 1/3)-1 = 3.71 Ω
Resistance for Z = (1/3 + 1/2 + 1/4)-1 = 0.923 Ω
Therefore, the combination of resistors in order of increasing resistance is Z X Y.

Example:
Referring to the circuit drawn, determine the value of I1, I and R, the combined resistance in the circuit.

E = I1 (160) = I2 (4000) = I3 (32000)

I1 = 2 / 160 = 0.0125 A
I2 = 2 / 4000 = 5 x 10-4 A
I3 = 2 / 32000 = 6.25 x 10-5 A

Since I = I1 + I2 + I3,
I = 13.1 mA

Applying Ohm‟s Law, R = 213.1 x 10-3 = 153 Ω


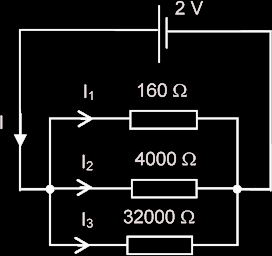Example:
A battery with an EMF of 20 V and an internal resistance of 2.0 Ω is connected to resistors R1 and R2 as shown in the diagram. A total current of 4.0 A is supplied by the battery and R2 has a resistance of 12 Ω. Calculate the resistance of R1 and the power supplied to each circuit component.

E - I r = I2 R2
20 – 4 (2) = I2 (12)
I2 = 1A

Therefore, I1 = 4 – 1 = 3 A

E – I r = I1 R1
12 = 3 R1
Therefore, R1 = 4

Power supplied to R1 = (I1)2 R1 = 36 W
Power supplied to R2 = (I2)2 R2 = 12 W

For potential divider with 2 resistors in series,

Potential drop across R1, V1 = R1 / (R1 + R2) x PD across R1 & R2
Potential drop across R2, V1 = R2 / (R1 + R2) x PD across R1 & R2

Example:
Two resistors, of resistance 300 kΩ and 500 kΩ respectively, form a potential divider with outer junctions maintained at potentials of +3 V and -15 V.

Determine the potential at the junction X between the resistors.

The potential difference across the 300 kΩ resistor = 300 / (300 + 500) [3 - (-15)] = 6.75 V

The potential at X = 3 - 6.75 = -3.75 V

A thermistor is a resistor whose resistance varies greatly with temperature. Its resistance decreases with increasing temperature. It can be used in potential divider circuits to monitor and control temperatures.

Example:
In the figure on the right, the thermistor has a resistance of 800 Ω when hot, and a resistance of 5000 Ω when cold. Determine the potential at W when the temperature is hot.

When thermistor is hot, potential difference across it

= [800 / (800 + 1700)] x (7 - 2)
= 1.6 V

The potential at W = 2 + 1.6 V = 3.6 V


A Light dependent resistor (LDR) is a resistor whose resistance varies with the intensity of light falling on it. Its resistance decreases with increasing light intensity. It can be used in a potential divider circuit to monitor light intensity.

Example:
In the figure below, the resistance of the LDR is 6.0 M in the dark but then drops to 2.0 k in the light. Determine the potential at point P when the LDR is in the light.

In the light the potential difference across the LDR

= [2k / (3k + 2k)] x (18 - 3)
= 6 V

The potential at P = 18 – 6= 12 V

The potential difference along the wire is proportional to the length of the wire. The sliding contact will move along wire AB until it finds a point along the wire such that the galvanometer shows a zero reading.

When the galvanometer shows a zero reading, the current through the galvanometer (and the device that is being tested) is zero and the potentiometer is said to be “balanced”.

If the cell has negligible internal resistance, and if the potentiometer is balanced,

EMF / PD of the unknown source, V = [L1 / (L1 + L2)] x E

Example:
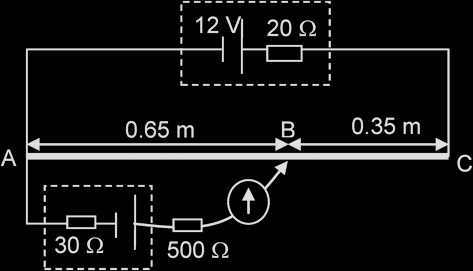In the circuit shown, the potentiometer wire has a resistance of 60 Ω. Determine the EMF of the unknown cell if the balanced point is at B.

Resistance of wire AB

= [0.65 / (0.65 + 0.35)] x 60
= 39 Ω

EMF of the test cell

= [39 / (60 + 20)] x 12
= 5.85 V